You are going to go through Probability Chapter 15 Extra Questions and Solutions For Class 10 CBSE Mathematics. This post presents to the students a clear conception of how to move with the basics of Extra Questions and answers. The expert prepared The Extra Questions and And Answers. https://cbsencertanswers.com/is very much to make things way simpler and easier for the students. Especially those who are appearing for the board exams. We took every care to make sure that the effort serves the purpose. So, let us find out Probability Chapter 15 Extra Questions and Solutions For Class 10 CBSE Mathematics. On this page, you can find Probability Chapter 15 Extra Questions and Solutions For Class 10 CBSE Mathematics.

1.The probability that it will foggy tomorrow is 0.85. What is the probability that it will not be foggy tomorrow?
Solution.
Probability that it will be foggy tomorrow P(E) = 0.85. Required to find, probability that it will not be foggy tomorrow P(E’). We know that sum of the probability of occurrence of an event and the probability of non-occurrence of an event is 1.
So,
P(E) + P(E’) = 1
0.85 + P(E’) = 1
P(E’) = 1 – 0.85
P(E’) = 0.15
Therefore, the probability that it will not be foggy tomorrow is = 0.15
2. Three coins are tossed together. Find the probability of getting:
(i) exactly two heads (ii) at most two heads
Solution
Three coins are tossed simultaneously. When three coins are tossed then the outcome will be anyone of these combinations.So, the possible outcomes are:
TTT, THT, TTH, THH. HTT, HHT, HTH, HHH.
So, the total number of outcomes is 8.
(i) For exactly two heads, the favourable outcome are THH, HHT, HTH
So, the total number of favourable outcomes is 3. We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting exactly two heads is 3/8.
(ii) For getting at least two heads the favourable outcomes are HHT, HTH, HHH, and THH
So, the total number of favourable outcomes is 4. We know that, Probability = Number of favourable outcomes/ Total number of outcomes.
Thus, the probability of getting at least two heads when three coins are tossed simultaneously = 4/8 = 1/2.
3. Two unbiased dice are thrown. Find the probability that the total of the numbers on the dice is greater than 10?
Solution.
Given A pair of dice is thrown. We have to find the probability that the total of numbers on the dice is greater than 10. First, let’s write the all possible events that can occur:
(1,1), (1,2), (1,3), (1,4), (1,5), (1,6), (2,1), (2,2), (2,3), (2,4), (2,5), (2,6),
(3,1), (3,2), (3,3), (3,4), (3,5), (3,6), (4,1), (4,2), (4,3), (4,4), (4,5), (4,6),
(5,1), (5,2), (5,3), (5,4), (5,5), (5,6), (6,1), (6,2), (6,3), (6,4), (6,5), (6,6).
It’s seen that the total number of events is 62 = 36 . Favourable events i.e. getting the total of numbers on the dice greater than 10 are (5, 6), (6, 5) and (6, 6). So, the total number of favourable events i.e. getting the total of numbers on the dice greater than 10 is 3.
We know that, Probability = Number of favourable outcomes/ Total number of outcomes. Thus, the probability of getting the total of numbers on the dice greater than 10 = 3/36 = 1/12.
4. A card is drawn at random from a pack of 52 cards. Find the probability that the card drawn is:
1) a black king 2) a jack, queen or a king 3) a black card 4) a red card
Solution.
A card is drawn at random from a pack of 52 cards. We have to find the probability of the following….
Total number of cards in a pack = 52.
- the probability of getting a black king = 2/52 = 1/26.
- A jack, queen or a king are 3 from each 4 suits.
So, the total number of a jack, queen and king are 12.
We know that, Probability = Number of favorable outcomes/ Total number of outcomes
Thus, the probability of getting a jack, queen or a king is 12/52 = 3/13
3. Total number of black cards in the pack is 26
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting black cards is 26/52 = 1/2.
4. Total number of red cards is 26
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting a red card = 26/52 = 1/2
5) An urn contains 10 red and 8 white balls. One ball is drawn at random. Find the probability that the ball drawn is white?
Solution.
It is given that an urn contains 10 red and 8 white balls. It is required to find probability that one ball is drawn at random and getting a white ball.
Total number of balls 10 + 8 = 18. Total number of white balls is 8.
We know that, Probability = Number of favorable outcomes/ Total number of outcomes.
Therefore, the probability of drawing a white ball from the urn is 8/18 = 4/9.
6) What is the probability that a number selected from the numbers 1, 2, 3, …, 15 is a multiple of 4?
Solution.
It is given that the numbers are from 1 to 15. One number is selected randomly.
We need to find the probability that the selected number is a multiple of 4
Total number between from 1 to 15 to 15. Numbers that are multiple of 4 are 4, 8 and 12. We know that, Probability = Number of favourable outcomes/ Total number of outcomes.
Thus, the probability of selecting a number which a multiple of 4 is 3/15 = 1/5.
7) In a lottery there are 10 prizes and 25 blanks. What is the probability of getting a prize?
Solution:
It is given that in a lottery there are 10 prizes and 25 blanks. We need to find probability of winning a prize
Total number of tickets is 10 + 25 = 35
Total number of prize carrying tickets is 10
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of winning a prize = 10/35 = 2/7.
8) A game of chance consists of spinning an arrow which is equally likely to come to rest pointing to one of the number, 1, 2, 3, …., 12 as shown in figure. What is the probability that it will point to?
1.A Prime number 2.An Odd number
Solution.
1. Favorable outcomes i.e. to get an odd number are 1, 3, 5, 7, 9, and 11
So, total number of favourable outcomes i.e. to get a prime number is 6
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting a prime number = 6/12 = 1/2 .
2. Favourable outcomes i.e. to get an even number are 2, 4, 6, 8, 10, and 12
So, total number of favourable outcomes i.e. to get an even number is 6
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting an even number = 6/12 = 1/2 .
9. Why is tossing a coin considered to be a fair way of deciding which team should choose ends in a game of cricket?
Solution:
No. of possible outcomes while tossing a coin = 2 i.e., 1 head or 1 tail
Probability = Number of favourable outcomes / Total number of outcomes,
P (getting head) = 1/2
P (getting tail) = 1/2
As we can see that the probability of both the events are equal, these are called equally like events.
Thus, tossing a coin is considered to be a fair way of deciding which team should choose ends in a game of cricket.
10. What is the probability that a number selected at random from the number 1, 2, 2, 3, 3, 3, 4, 4, 4, 4 will be their average?
Solution.
Given numbers are 1, 2, 2, 3, 3, 3, 4, 4, 4, 4 . Total number of possible outcomes = 10
Average of the numbers = ( 1+ 2 + 3 + 3 + 3 + 4 + 4 + 4 + 4 ) / 10 = 30/10 = 3.
Now, let E be the event of getting 3.
Number of favourable outcomes = 3 {3, 3, 3}
P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 3/10
Therefore, the probability that a number selected at random will be the average is 3/10.
11. A bag contains 8 red, 6 white and 4 black balls. A ball is drawn at random from the bag. Find the probability that the drawn ball is :
(i) Red or white (ii) Neither white nor black
Solution.
Total number of balls = 8 + 6 + 4 = 18. Total no. of possible outcomes = 18.
i) Let E = Event of getting red or white ball
No. of favourable outcomes = 14 ( 8 red balls + 6 white balls)
Probability, P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 14/18
P(E) = 7/9.
ii) Let E = event of getting neither a white nor a black ball
No. of favourable outcomes = 18 – 6 – 4
= 8 (Total balls – no. of white balls – no. of black balls)
Probability, P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 8/18 = 4/9.
12) Find the probability that a number selected at random from the numbers 1, 2, 3…. 35 is :
(i) Prime number (ii) Multiple of 7
Solution.
Numbers from 1, 2, 3….. 35 are a total of 35. Total no. of possible outcomes = 35.
(i) Let E = event of getting a prime number
No. of favorable outcomes = 11 {2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31)
Probability, P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 11/35.
(ii) Let E = event of getting a number which is a multiple of 7
No. of favourable outcomes = 5 {7, 14, 21, 28, 35}
P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 5/35 = 1/7.
13) A lot consists of 144 ball pens of which 20 are defective and others good. Nomii will buy a pen if it is good, but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that she will buy it ?
Solution.
No. of good pens = 144 – 20 = 124. No. of detective pens = 20 . Total no. of possible outcomes =144 (total no. of pens).
So, for her to buy it the pen should be a good one. let E = event of buying a pen which is good.
No. of favourable outcomes = 124 (124 good pens)
P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 124/144 = 31/36 .
So, the probability that Nomii will buy the pen is 31/36 .
14) 12 defective pens are accidently mixed with 132 good ones. It is not possible to just look at pen and tell whether or not it is defective. One pen is taken out at random from this lot. Determine the probability that the pen taken out is good one?
Solution.
We have,
No. of good pens = 132. No. of defective pens = 12 . So, the total no. of pens = 132 + 12 = 144 .
Then, the total no. of possible outcomes = 144.
Now, let E = event of getting a good pen.
No. of favorable out comes = 132 {132 good pens}
P(E) = Number of favorable outcomes / Total number of outcomes
P(E) = 132/144 = 11/12.
15) A bag contains lemon flavoured candies only. Mahi takes out one candy without looking into the bag. What is the probability that she takes out:
(i) an orange flavored candy
(ii) a lemon flavored candy
Solution.
(i) an orange flavored candy
We know that the bag contains lemon flavored candies only. So, the event that Mahi will take out an orange flavored candy is an impossible event.
Thus, the probability of impossible event is 0 .
P(an orange flavored candy) = 0.
(ii) a lemon flavored candy
As the bag contains lemon flavored candies only. Then, the event that Mahi will take out a lemon flavoured candy is sure event. Thus, the probability of sure event is 1.
P(a lemon flavoured candy) = 1.
16) A bag contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is not red ?
Solution.
A bag contains 3 red and 5 black balls.
So, the total no. of possible outcomes = 8 (3 red + 5 black).
Let E = event of getting red ball.
No. of favourable outcomes = 3 (as there are 3 red)
P(E) = Number of favorable outcomes/ Total number of outcomes
P(E) = 3/8.
Now,
P(E) + P(E’) = 1
P(E’) = 1 – P(E)
P(E’)= 1 – 3/8
P(E’) = 5/8 .
So, 5/8 is the probability of not getting red balls.
17) A box contains 5 red marbles, 8 white marbles and 4 green marbles. One marble is taken out of the box at random. What is the probability that the marble taken out will be not green ?
Solution.
A box containing 5 red, 8 white and 4 green marbles. So, the total no. of possible outcomes = 17 (5 red + 8 white + 4 green).
Let E= event of getting a green marble
Number of favourable outcomes = 4 (as 4 green marbles)
Probability, P(E) = Number of favourable outcomes/ Total number of outcomes
P(E) = 4/17
So,
P(E) + P(E’) = 1
P(E’) = 1 – P(E)
P(E’)= 1 – 4/17
P(E’) = 13/17 .
Therefore, the probability that the marble taken out is not green is 13/17.
18) Suppose you drop a tie at random on the rectangular region shown in fig. below. What is the probability that it will land inside the circle with diameter 1 m?
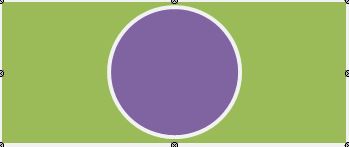
Solution.
Area of a circle with radius 0.5 m A circle = (0.5)2 = 0.25 πm2 . Area of rectangle = 3 x 2 = 6m2.
Probability ( Geometric ) = ( measured specific region ) / ( measured whole region).
Probability ( Geometric ) = ( area of the circle in violet )/ ( area of the rectangle in olive)
= 0.25 πm2 / 6m2.
= π / 24 .
Therefore, the probability that the tie will land inside the circle = π/24.
19 ) In a class, there are 18 girls and 16 boys. The class teacher wants to choose one pupil for class monitor. What she does, she writes the name of each pupil on a card and puts them into a basket and mixes thoroughly. A child is asked to pick one card from the basket. What is the probability that the name written on the card is the name of a girl ?
Solution.
In a class there are 18 girls and 16 boys, the class teacher wants to choose one name. The class teacher writes all pupils’ name on a card and puts them in basket and mixes well thoroughly. A child picks one card. It is required to find the probability that the name written on the card is a girl’s name …….
Total number of students in the class = 18 + 16 = 34.
The names of a girl are 18, so the number of favourable cases is 18
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting a name of girl on the card = 18/34 = 9/17.
20) One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting a king of red suit ?
Solution.
It is given that one card is drawn from a well shuffled deck of 52 playing cards.We have to find the probability of a king in red suit. Total number of cards is 52
Total number of cards which are king of red suit is 2
Number of favourable outcomes i.e. Total number of cards which are king of red suit is 2.
We know that, Probability = Number of favourable outcomes/ Total number of outcomes
Thus, the probability of getting cards which is a king of red suit = 2/52 = 1/26.